tahrir
Dekart koordinatalar sistemasi
tahrir
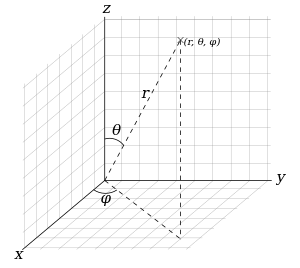
Agar nuqtaning sferik koordinatalari
(
r
,
θ
,
φ
)
{\displaystyle (r,\;\theta ,\;\varphi )}
{
x
=
r
sin
θ
cos
φ
,
y
=
r
sin
θ
sin
φ
,
z
=
r
cos
θ
.
{\displaystyle {\begin{cases}x=r\sin \theta \cos \varphi ,\\y=r\sin \theta \sin \varphi ,\\z=r\cos \theta .\end{cases}}}
Dekart koordinatalaridan sferik koordinatalarga oʻtish uchun esa:
{
r
=
x
2
+
y
2
+
z
2
,
θ
=
arccos
z
x
2
+
y
2
+
z
2
=
a
r
c
t
g
x
2
+
y
2
z
,
φ
=
a
r
c
t
g
y
x
.
{\displaystyle {\begin{cases}r={\sqrt {x^{2}+y^{2}+z^{2}}},\\\theta =\arccos {\dfrac {z}{\sqrt {x^{2}+y^{2}+z^{2}}}}=\mathrm {arctg} {\dfrac {\sqrt {x^{2}+y^{2}}}{z}},\\\varphi =\mathrm {arctg} {\dfrac {y}{x}}.\end{cases}}}
Sferik koordinatalarga oʻtish yakobiani :
J
=
∂
(
x
,
y
,
z
)
∂
(
r
,
θ
,
φ
)
=
|
sin
θ
cos
φ
r
cos
θ
cos
φ
−
r
sin
θ
sin
φ
sin
θ
sin
φ
r
cos
θ
sin
φ
r
sin
θ
cos
φ
cos
θ
−
r
sin
θ
0
|
=
=
cos
θ
(
r
2
cos
φ
2
cos
θ
sin
θ
+
r
2
sin
2
φ
cos
θ
sin
θ
)
+
r
sin
θ
(
r
sin
2
θ
cos
2
φ
+
r
sin
2
θ
sin
2
φ
)
=
=
r
2
cos
2
θ
sin
θ
+
r
2
sin
2
θ
sin
θ
=
=
r
2
sin
θ
.
{\displaystyle {\begin{alignedat}{2}J&={\frac {\partial (x,y,z)}{\partial (r,\theta ,\varphi )}}={\begin{vmatrix}\sin \theta \cos \varphi &r\cos \theta \cos \varphi &-r\sin \theta \sin \varphi \\\sin \theta \sin \varphi &r\cos \theta \sin \varphi &r\sin \theta \cos \varphi \\\cos \theta &-r\sin \theta &0\end{vmatrix}}=\\&=\cos \theta (r^{2}\cos \varphi ^{2}\cos \theta \sin \theta +r^{2}\sin ^{2}\varphi \cos \theta \sin \theta )+r\sin \theta (r\sin ^{2}\theta \cos ^{2}\varphi +r\sin ^{2}\theta \sin ^{2}\varphi )=\\&=r^{2}\cos ^{2}\theta \sin \theta +r^{2}\sin ^{2}\theta \sin \theta =\\&=r^{2}\sin \theta .\end{alignedat}}}
Shunday qilib, dekart koordinatalaridan sferik koordinatalarga oʻtishdagi hajm elementi quyidagi koʻrinishga ega boʻladi:
d
V
=
d
x
d
y
d
z
=
J
(
r
,
θ
,
φ
)
d
r
d
θ
d
φ
=
r
2
sin
θ
d
r
d
θ
d
φ
{\displaystyle \mathrm {d} V=\mathrm {d} x\,\mathrm {d} y\,\mathrm {d} z=J(r,\theta ,\varphi )\,\mathrm {d} r\,\mathrm {d} \theta \,\mathrm {d} \varphi =r^{2}\sin \theta \,\,\mathrm {d} r\,\mathrm {d} \theta \,\mathrm {d} \varphi }
Silindrik koordinatalar sistemasi
tahrir
Agar nuqtaning silindrik koordinatalari berilgan boʻlsa, sferik koordinatalarga oʻtish uchun quyidagi formulalardan foydalaniladi:
{
ρ
=
r
sin
θ
φ
=
φ
z
=
r
cos
θ
{\displaystyle {\begin{cases}\rho =r\sin \theta \\\varphi =\varphi \\z=r\cos \theta \end{cases}}}
Yoki aksincha, sferik koordinatalardan silindrik koordinatalarga oʻtish uchun quyidagi formulalardan foydalaniladi:
{
r
=
ρ
2
+
z
2
,
θ
=
a
r
c
t
g
ρ
z
,
φ
=
φ
.
{\displaystyle {\begin{cases}r={\sqrt {\rho ^{2}+z^{2}}},\\\theta =\mathrm {arctg} {\dfrac {\rho }{z}},\\\varphi =\varphi .\end{cases}}}
Silindrik koordinatalardan sferik koordinatalarga oʻtish yakobiani :
J
=
r
{\displaystyle J=r}
Sferik koordinatalar sistemasida differensiallash va integrallash
tahrir
(
r
,
θ
,
φ
)
{\displaystyle (r,\theta ,\varphi )}
(
r
+
d
r
,
θ
+
d
θ
,
φ
+
d
φ
)
{\displaystyle (r+\mathrm {d} r,\,\theta +\mathrm {d} \theta ,\,\varphi +\mathrm {d} \varphi )}
d
r
{\displaystyle \mathrm {d} \mathbf {r} }
d
r
=
d
r
r
^
+
r
d
θ
θ
^
+
r
sin
θ
d
φ
φ
^
,
{\displaystyle \mathrm {d} \mathbf {r} =\mathrm {d} r\,{\boldsymbol {\hat {r}}}+r\,\mathrm {d} \theta \,{\boldsymbol {\hat {\theta }}}+r\sin {\theta }\,\mathrm {d} \varphi \,\mathbf {\boldsymbol {\hat {\varphi }}} ,}
bu yerda
r
^
=
sin
θ
cos
φ
ı
^
+
sin
θ
sin
φ
ȷ
^
+
cos
θ
k
^
{\displaystyle {\boldsymbol {\hat {r}}}=\sin \theta \cos \varphi {\boldsymbol {\hat {\imath }}}+\sin \theta \sin \varphi {\boldsymbol {\hat {\jmath }}}+\cos \theta {\boldsymbol {\hat {k}}}}
θ
^
=
cos
θ
cos
φ
ı
^
+
cos
θ
sin
φ
ȷ
^
−
sin
θ
k
^
{\displaystyle {\boldsymbol {\hat {\theta }}}=\cos \theta \cos \varphi {\boldsymbol {\hat {\imath }}}+\cos \theta \sin \varphi {\boldsymbol {\hat {\jmath }}}-\sin \theta {\boldsymbol {\hat {k}}}}
φ
^
=
−
sin
φ
ı
^
+
cos
φ
ȷ
^
{\displaystyle {\boldsymbol {\hat {\varphi }}}=-\sin \varphi {\boldsymbol {\hat {\imath }}}+\cos \varphi {\boldsymbol {\hat {\jmath }}}}
Sferik koordinatalar ortogonal hisoblanadi. Shu sababli ularning metrik tenzori diagonal koʻrinishda boʻladi[ 1]
g
i
j
=
(
1
0
0
0
r
2
0
0
0
r
2
sin
2
θ
)
,
g
i
j
=
(
1
0
0
0
1
r
2
0
0
0
1
r
2
sin
2
θ
)
{\displaystyle g_{ij}={\begin{pmatrix}1&0&0\\0&r^{2}&0\\0&0&r^{2}\sin ^{2}\theta \end{pmatrix}},\quad g^{ij}={\begin{pmatrix}1&0&0\\0&{\dfrac {1}{r^{2}}}&0\\0&0&{\dfrac {1}{r^{2}\sin ^{2}\theta }}\end{pmatrix}}}
det
(
g
i
j
)
=
r
4
sin
2
θ
.
{\displaystyle \det(g_{ij})=r^{4}\sin ^{2}\theta .\ }
Yoy uzunligi differensialining kvadrati:
d
s
2
=
d
r
2
+
r
2
d
θ
2
+
r
2
sin
2
θ
d
φ
2
.
{\displaystyle ds^{2}=dr^{2}+r^{2}\,d\theta ^{2}+r^{2}\sin ^{2}\theta \,d\varphi ^{2}.}
H
r
=
1
,
H
θ
=
r
,
H
φ
=
r
sin
θ
.
{\displaystyle H_{r}=1,\quad H_{\theta }=r,\quad H_{\varphi }=r\sin \theta .}
Γ
22
1
=
−
r
,
Γ
33
1
=
−
r
sin
2
θ
,
{\displaystyle \Gamma _{22}^{1}=-r,\quad \Gamma _{33}^{1}=-r\sin ^{2}\theta ,}
Γ
21
2
=
Γ
12
2
=
Γ
13
3
=
Γ
31
3
=
1
r
,
{\displaystyle \Gamma _{21}^{2}=\Gamma _{12}^{2}=\Gamma _{13}^{3}=\Gamma _{31}^{3}={\frac {1}{r}},}
Γ
33
2
=
−
cos
θ
sin
θ
,
Γ
23
3
=
Γ
32
3
=
c
t
g
θ
.
{\displaystyle \Gamma _{33}^{2}=-\cos \theta \sin \theta ,\quad \Gamma _{23}^{3}=\Gamma _{32}^{3}=\mathrm {ctg} \,\theta .}
Sferik koordinatalar sistemasida birlik vektorlar Sferik koordinatalar sistemasida masofa
tahrir
Fazodagi vaziyati sferik koordinatalar sistemasida berilgan ikki nuqtaning joylashuvi quyidagicha boʻlsin:
r
=
(
r
,
θ
,
φ
)
,
r
′
=
(
r
′
,
θ
′
,
φ
′
)
{\displaystyle {\begin{aligned}{\mathbf {r} }&=(r,\theta ,\varphi ),\\{\mathbf {r} '}&=(r',\theta ',\varphi ')\end{aligned}}}
U holda ushbu nuqtalar orasidagi masofani quyidagi formula orqali hisoblash mumkin:
D
=
r
2
+
r
′
2
−
2
r
r
′
(
sin
θ
sin
θ
′
cos
(
φ
−
φ
′
)
+
cos
θ
cos
θ
′
)
{\displaystyle {\begin{aligned}{\mathbf {D} }&={\sqrt {r^{2}+r'^{2}-2rr'(\sin {\theta }\sin {\theta '}\cos {(\varphi -\varphi ')}+\cos {\theta }\cos {\theta '})}}\end{aligned}}}
Nuqtaning vaziyati sferik koordinatalarda quyidagi koʻrinishda berilgan boʻlsin:
r
=
r
r
^
.
{\displaystyle \mathbf {r} =r\mathbf {\hat {r}} .}
U holda uning tezligi:
v
=
r
˙
r
^
+
r
θ
˙
θ
^
+
r
φ
˙
sin
θ
φ
^
,
{\displaystyle \mathbf {v} ={\dot {r}}\mathbf {\hat {r}} +r\,{\dot {\theta }}\,{\hat {\boldsymbol {\theta }}}+r\,{\dot {\varphi }}\sin \theta \,\mathbf {\hat {\boldsymbol {\varphi }}} ,}
hamda tezlanishi:
a
=
(
r
¨
−
r
θ
˙
2
−
r
φ
˙
2
sin
2
θ
)
r
^
+
(
r
θ
¨
+
2
r
˙
θ
˙
−
r
φ
˙
2
sin
θ
cos
θ
)
θ
^
+
(
r
φ
¨
sin
θ
+
2
r
˙
φ
˙
sin
θ
+
2
r
θ
˙
φ
˙
cos
θ
)
φ
^
.
{\displaystyle {\begin{aligned}\mathbf {a} ={}&\left({\ddot {r}}-r\,{\dot {\theta }}^{2}-r\,{\dot {\varphi }}^{2}\sin ^{2}\theta \right)\mathbf {\hat {r}} \\&{}+\left(r\,{\ddot {\theta }}+2{\dot {r}}\,{\dot {\theta }}-r\,{\dot {\varphi }}^{2}\sin \theta \cos \theta \right){\hat {\boldsymbol {\theta }}}\\&{}+\left(r{\ddot {\varphi }}\,\sin \theta +2{\dot {r}}\,{\dot {\varphi }}\,\sin \theta +2r\,{\dot {\theta }}\,{\dot {\varphi }}\,\cos \theta \right){\hat {\boldsymbol {\varphi }}}.\end{aligned}}}
ga teng boʻladi.
Burchak momenti :
L
=
m
r
×
v
=
m
r
2
(
θ
˙
φ
^
−
φ
˙
sin
θ
θ
^
)
.
{\displaystyle \mathbf {L} =m\mathbf {r} \times \mathbf {v} =mr^{2}({\dot {\theta }}\,{\hat {\boldsymbol {\varphi }}}-{\dot {\varphi }}\sin \theta \,\mathbf {\hat {\boldsymbol {\theta }}} ).}
φ
{\displaystyle \varphi }
θ
=
π
2
{\displaystyle \theta ={\frac {\pi }{2}}}
qutb koordinatalar sistemasiga oʻtadi.
L
=
−
i
ℏ
r
×
∇
=
i
ℏ
(
θ
^
sin
(
θ
)
∂
∂
ϕ
−
ϕ
^
∂
∂
θ
)
.
{\displaystyle \mathbf {L} =-i\hbar ~\mathbf {r} \times \nabla =i\hbar \left({\frac {\hat {\boldsymbol {\theta }}}{\sin(\theta )}}{\frac {\partial }{\partial \phi }}-{\hat {\boldsymbol {\phi }}}{\frac {\partial }{\partial \theta }}\right).}
Morse PM , Feshbach H . Methods of Theoretical Physics, Part I . New York: McGraw-Hill, 1953 — 658 -bet. ISBN 0-07-043316-X . Margenau H , Murphy GM. The Mathematics of Physics and Chemistry . New York: D. van Nostrand, 1956 — 177–178 -bet. Korn GA, Korn TM . Mathematical Handbook for Scientists and Engineers . New York: McGraw-Hill, 1961 — 174–175-bet. ASIN B0000CKZX7. Sauer R, Szabó I. Mathematische Hilfsmittel des Ingenieurs . New York: Springer Verlag, 1967 — 95–96-bet. Moon P, Spencer DE „Spherical Coordinates (r, θ, ψ)“, . Field Theory Handbook, Including Coordinate Systems, Differential Equations, and Their Solutions , corrected 2nd ed., 3rd print, New York: Springer-Verlag, 1988 — 24–27 (Table 1.05)-bet. ISBN 978-0-387-18430-2 . Duffett-Smith P, Zwart J. Practical Astronomy with your Calculator or Spreadsheet, 4th Edition . New York: Cambridge University Press, 2011 — 34 -bet. ISBN 978-0521146548 . Python tilida yozilgan programma
↑ https://archive.org/details/mathematicsofphy0002marg